For students, math can seem like an impenetrable puzzle. While teachers may be fascinated with an equation’s clarity and simplicity, students may be counting down the minutes till noon. The math problems are not difficult to solve if the right assistance is provided. However, connecting the dots for students between mathematical assignments and real life is one of the most enjoyable aspects of teaching math. The right solving techniques can make mathematics more fun. And notably, quadratic equations are an excellent area to explore by adding tools to your equation-solving kit and utilizing equations to model real-world structures and events.
In mathematics, solving quadratic equations is a crucial skill. Quadratic equations can be used to model a variety of events in life. Different approaches can be used to solve a quadratic equation, and the most relevant method can only be determined by looking at the provided equation. Quadratic equations will almost certainly appear on the SAT and ACT. However, they can be difficult to fix, especially when there are several approaches you might use.
When you make the transition from linear to higher-order equations, it may be quite an adventure. Equations that are unusual, solution sets that are odd, and results that are less obvious might make things more difficult. Quadratic equations require students to perform a series of unique and interesting things with mathematics, which is fortunate. Many students find it difficult to solve algebra problems and seek professional math help. The internet is loaded with queries like ‘who will do my math homework’, or ‘solve quadratic equations’, and professionals respond to such queries and provide academic assistance.
What Are Quadratic Equations?

Any equation that can be stated in the form ax2 + bx + c = 0, in which variable x is to be solved, a, b, and c are the numbers, and a is not 0, is a quadratic equation. Such an equation is a quadratic equation because of the squared term (ax2). Finding any values for x where the equation goes to 0 is what solving a quadratic equation entails. Quadratic equations can have two, one, or no answers. The quadratic equation simplifies to 0 if x is replaced with a member of the solution set. A quadratic equation is a single-variable polynomial equation with the maximum exponent of the variable equal to 2.
Because quadratic equations are used to calculate parabolas or arcs, they have a wide range of real-world applications. The quadratic equation is used in construction projects like bridges to calculate the arc of the structure, and even roller coasters use quadratics to develop adrenaline-pumping tracks. Quadratics are even used in famous computer games where the quadratic formula is used to determine the arc of the characters doing acts.
Solving Quadratic Equations & The Math Helpers
As for math helpers, it’s critical to deliver information properly and expose students to the many cues they can encounter. Solutions, solution sets, roots, zeros, and xx-intercepts may be requested from students. It might be rather overwhelming for students to come upon all of these phrases without any prior preparation! Take the time to explain what each word signifies and how prompts will be delivered to learners. Students must understand that the x-intercepts are the roots and zeros of a quadratic equation. When you solve a quadratic equation, you’re looking for the roots, also known as the xx-intercepts. You must also specify the format in which you intend students to submit solution sets. Remember that vocabulary that professionals, as math helpers, take for granted can be intimidating to students.
FOUR WAYS OF SOLVING QUADRATIC EQUATIONS
A complex quadratic equation can be easily solved using four methods: by completing the square method, obtaining the square root, factoring, and using the quadratic formula. Notably, factoring and calculating the square root only work in particular instances. Getting the square root of a quadratic equation entails taking the square root of each side of the equation. Factorization, on the other hand, involves division of the quadratic equation into two equal-valued linear equations. The quadratic formula and completing the square, on the other hand, will allow you to solve any quadratic equation using their respective methods.
Factoring Quadratic Equations to Solve Them
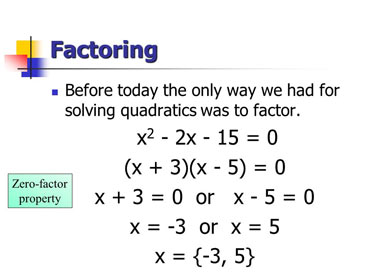
This is the most widely used method for solving quadratic equations. When a = 1 or when a is not equal to 1, this approach shows you how to solve quadratic equations of the type ax2 + bx + c = 0. It’s easy to understand when a = 1. When a equals 1, things get a little repetitive. You’ll have to depend on trial and error techniques. Factoring is a method of solving quadratic equations that needs some analysis. The goal is to find pairs of numbers that will multiply to generate c and add to produce b when multiplied together. You’ll have two binomials if you find the appropriate combination, each of which can be solved independently.
Completing Square Method
The famous completing square method can be used to solve all quadratic equations. You can make a perfect square on the left side of the equation by altering your constant (c). You can factor a perfect square into two identical binomials, which you can use to solve for any valid x number. The more students grasp factoring, the easier it will be to answer quadratic equations, and the faster they will be able to solve more complicated quadratic equations. If given the opportunity to utilize the quadratic equation every time, be aware that some students may try to avoid factoring entirely. And allowing kids to skip factoring will negatively impact them in the long term.
The “Square Root” Method
When your bx term is 0, you can utilize the square root approach. The constant (c) is moved to the right side of the equal sign, both sides of the equation are divided by a, and both sides of the equation are squared. For x, you’ll have two options (one positive and one negative). To solve quadratic equations, place the square on one side and the constant on the other if the quadratic equation has a square and a constant (no first-degree term). Then just multiply both equations’ sides by the square root. This is one of the quickest methods used for solving quadratic equations.
The Quadratic Formula
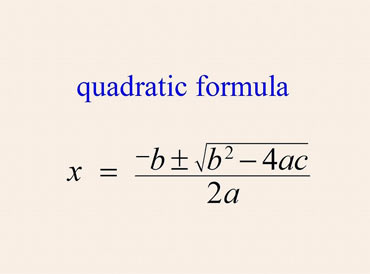
The quadratic formula is a complex plug and play equation that allows you to put values for a, b, and c and then solve the problem for any x values that appear. The quadratic formula can be used to solve any quadratic equation of the form ax2 + bx + c = 0, in which a is not equal to zero and can be solved for both real and imaginary solutions. All quadratic equations, whether their solutions are rational or irrational, real or complex, can be solved using this method.
When the positive sign in front of the square root is chosen, the quadratic formula yields two roots, one when the positive sign is chosen and the other when the negative sign is chosen. There are three possibilities:
- The roots will be unequal and real if the discriminant is positive.
- If the discriminant is equal to 0, then the two roots are real & equal.
- The roots will be complex if the discriminant is negative, and each root will be the complex conjugate of the other root.
Developing Techniques for A Module on Quadratic Equations
Always start your lesson preparation with learning objectives! Learning goals, as previously said, help students see meaning in everything they do, especially if you take the time to specify which learning goal you are working on with specific tasks and assignments. Creating learning goals first ensures that your assessments are aligned with your objectives. Learning objectives should, of course, be based on the standards.
A lesson on quadratic equations offers plenty of opportunities for application and different assessments. Students benefit from technical challenges because they help them see the link between mathematical principles and real-life situations. Students who generally struggle on written tests gain confidence from alternative assessments. A good learning technique can help students master the art of solving quadratic equations and solving algebra problems.
Final Words
Quadratics are defined as a polynomial equation of the second degree, which means it contains at least one squared term. Quadratic equations is another name for it. The quadratic equation has the generic form ax2 + bx + c = 0, where x is an unknown variable and a, b, and c are numerical coefficients. It is obvious that solving quadratic equations is difficult for students. The completing square method, factorization, square root, and quadratic formula are some of the most extensively used methods for obtaining solutions to quadratic equations. These methods help students follow a predefined method and apply it. Professional math helpers guide students and assist them with their algebra problems and quadratic equations when students find it difficult to solve them on their own. Many students seek help from professionals and ace their academic scores with the right professional academic assistance.